Physics For Scientists And Engineers 6E - part 45

59.

The pilot of an airplane executes a constant-speed loop-the-
loop maneuver in a vertical circle. The speed of the airplane
is 300 mi/h, and the radius of the circle is 1 200 ft. (a) What
is the pilot’s apparent weight at the lowest point if his true
weight is 160 lb? (b) What is his apparent weight at the
highest point? (c) What If? Describe how the pilot could ex-
perience weightlessness if both the radius and the speed can
be varied. (Note: His apparent weight is equal to the magni-
tude of the force exerted by the seat on his body.)

60.

A penny of mass 3.10 g rests on a small 20.0-g block sup-
ported by a spinning disk (Fig. P6.60). The coefficients of
friction between block and disk are 0.750 (static) and

above the horizontal. If the radius of the tub is 0.330 m,
what rate of revolution is needed?

51. We will study the most important work of Nobel laureate

Arthur  Compton  in  Chapter  40.  Disturbed  by  speeding
cars outside the physics building at Washington University
in St. Louis, Compton designed a speed bump and had it
installed. Suppose that a 1 800-kg car passes over a bump
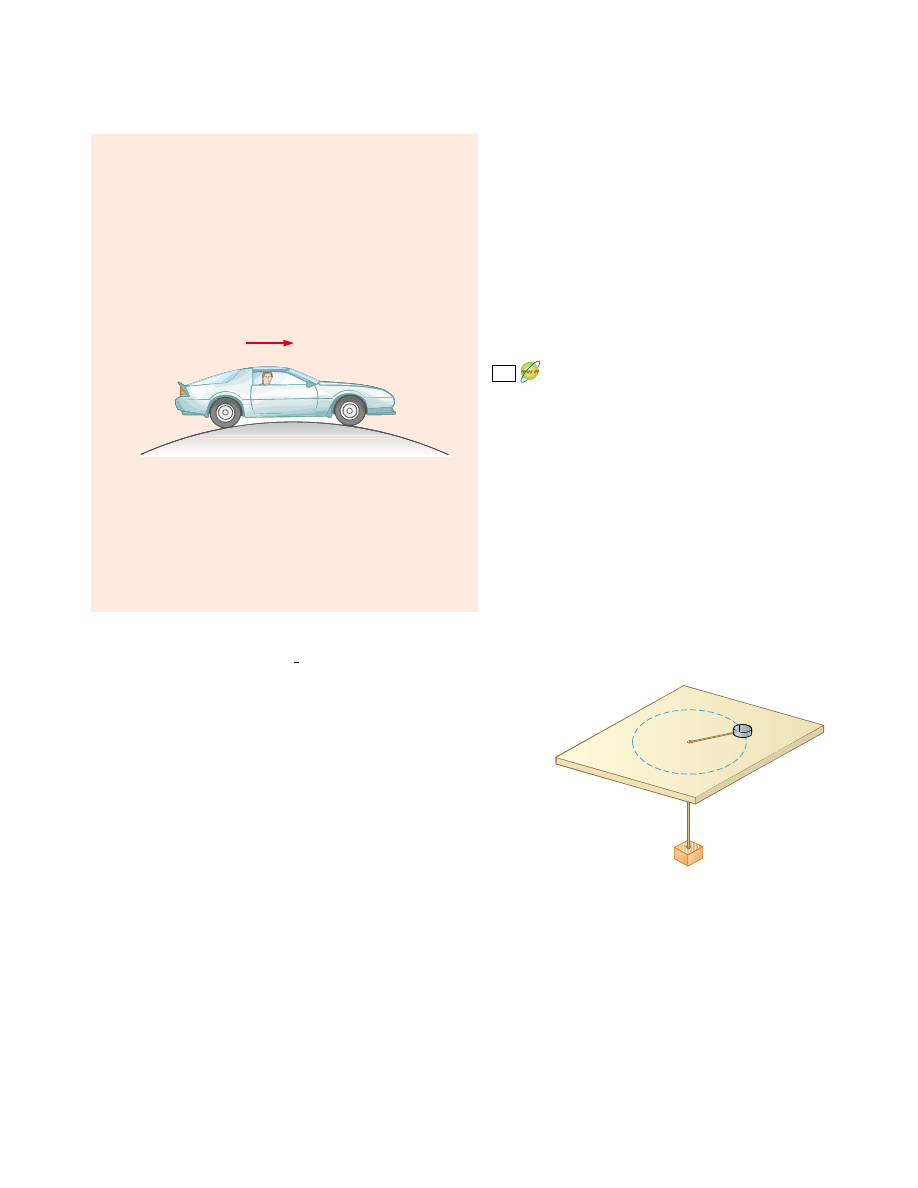
in  a  roadway  that  follows  the  arc  of  a  circle  of  radius
20.4 m as in Figure P6.51. (a) What force does the road ex-
ert  on  the  car  as  the  car  passes  the  highest  point  of  the
bump if the car travels at 30.0 km/h? (b) What If? What is
the maximum speed the car can have as it passes this high-
est point without losing contact with the road?

52.

A car of mass m passes over a bump in a road that follows
the arc of a circle of radius R as in Figure P6.51. (a) What
force does the road exert on the car as the car passes the
highest point of the bump if the car travels at a speed v?
(b) What If? What is the maximum speed the car can have
as it passes this highest point without losing contact with
the road?

53.

Interpret the graph in Figure 6.18(b). Proceed as follows:
(a) Find the slope of the straight line, including its units.
(b) From Equation 6.6,

, identify the theoreti-

cal slope of a graph of resistive force versus squared speed.
(c) Set the experimental and theoretical slopes equal to
each other and proceed to calculate the drag coefficient of
the filters. Use the value for the density of air listed on the
book’s endpapers. Model the cross-sectional area of the fil-
ters as that of a circle of radius 10.5 cm. (d) Arbitrarily
choose the eighth data point on the graph and find its ver-
tical separation from the line of best fit. Express this scat-
ter as a percentage. (e) In a short paragraph state what the
graph demonstrates and compare it to the theoretical pre-
diction. You will need to make reference to the quantities
plotted on the axes, to the shape of the graph line, to the
data points, and to the results of parts (c) and (d).

54.

A  student  builds  and  calibrates  an  accelerometer,  which
she uses to determine the speed of her car around a cer-
tain  unbanked  highway  curve.  The  accelerometer  is  a
plumb bob with a protractor that she attaches to the roof
of her car. A friend riding in the car with her observes that
the plumb bob hangs at an angle of 15.0° from the vertical
when the car has a speed of 23.0 m/s. (a) What is the cen-
tripetal  acceleration  of  the  car  rounding  the  curve?
(b) What is the radius of the curve? (c) What is the speed
of the car if the plumb bob deflection is 9.00° while round-
ing the same curve?

55.

Suppose the boxcar of Figure 6.13 is moving with constant
acceleration a up a hill that makes an angle 1 with the

R !

D-Av

horizontal. If the pendulum makes a constant angle " with
the perpendicular to the ceiling, what is a?

56.

(a) A luggage carousel at an airport has the form of a sec-
tion of a large cone, steadily rotating about its vertical axis.
Its  metallic  surface  slopes  downward  toward  the  outside,
making  an  angle  of  20.0° with  the  horizontal.  A  piece  of
luggage  having  mass  30.0 kg  is  placed  on  the  carousel,
7.46 m  from  the  axis  of  rotation.  The  travel  bag  goes
around once in 38.0 s. Calculate the force of static friction
between the bag and the carousel. (b) The drive motor is
shifted  to  turn  the  carousel  at  a  higher  constant  rate  of
rotation,  and  the  piece  of  luggage  is  bumped  to  another
position,  7.94 m  from  the  axis  of  rotation.  Now  going
around once in every 34.0 s, the bag is on the verge of slip-
ping.  Calculate  the  coefficient  of  static  friction  between
the bag and the carousel.

Because the Earth rotates about its axis, a point on

the equator experiences a centripetal acceleration of
0.033 7 m/s

, while a point at the poles experiences no

centripetal acceleration. (a) Show that at the equator the
gravitational force on an object must exceed the normal
force required to support the object. That is, show that the
object’s true weight exceeds its apparent weight. (b) What
is the apparent weight at the equator and at the poles of a
person having a mass of 75.0 kg? (Assume the Earth is a
uniform sphere and take g ! 9.800 m/s

58.

An air puck of mass m

is tied to a string and allowed to re-

volve in a circle of radius R on a frictionless horizontal
table. The other end of the string passes through a hole in
the center of the table, and a counterweight of mass m

tied to it (Fig. P6.58). The suspended object remains in
equilibrium while the puck on the tabletop revolves. What
is (a) the tension in the string? (b) the radial force acting
on the puck? (c) the speed of the puck?

57.

Problems

177

Figure P6.58

Figure P6.51 Problems 51 and 52.

178

CHAPTE R 6 • Circular Motion and Other Applications of Newton’s Laws

Figure P6.65

(a) Find the speed of a point on the rim of the wheel in
terms of the acceleration due to gravity and the radius R of
the wheel. (b) If the mass of the putty is m, what is the
magnitude of the force that held it to the wheel?
An amusement park ride consists of a large vertical cylin-
der that spins about its axis fast enough such that any per-
son inside is held up against the wall when the floor drops
away (Fig. P6.65). The coefficient of static friction between
person and wall is #

, and the radius of the cylinder is R.

(a) Show that the maximum period of revolution neces-
sary to keep the person from falling is T ! (4/

/g)

1/2

(b) Obtain a numerical value for T if R ! 4.00 m and
#

0.400. How many revolutions per minute does the

cylinder make?

65.

8.00 m

2.50 m

Figure P6.63

Block

Disk

Penny

12.0 cm

Figure P6.60

Figure P6.61

Color Box/Getty Images

0.640 (kinetic) while those for the penny and block are
0.520 (static) and 0.450 (kinetic). What is the maximum
rate of rotation in revolutions per minute that the disk can
have, without the block or penny sliding on the disk?

61.

Figure  P6.61  shows  a  Ferris  wheel  that  rotates  four  times
each minute. It carries each car around a circle of diame-
ter  18.0 m.  (a)  What  is  the  centripetal  acceleration  of  a
rider?  What  force  does  the  seat  exert  on  a  40.0-kg  rider
(b) at  the  lowest  point  of  the  ride  and  (c)  at  the  highest
point  of  the  ride?  (d)  What  force  (magnitude  and  direc-
tion)  does  the  seat  exert  on  a  rider  when  the  rider  is
halfway between top and bottom?

62.

A space station, in the form of a wheel 120 m in diameter,
rotates to provide an “artificial gravity” of 3.00 m/s

for

persons who walk around on the inner wall of the outer
rim. Find the rate of rotation of the wheel (in revolutions
per minute) that will produce this effect.

63.

An  amusement  park  ride  consists  of  a  rotating  circular
platform  8.00 m  in  diameter  from  which  10.0-kg  seats
are suspended  at  the  end  of  2.50-m  massless  chains
(Fig. P6.63). When the system rotates, the chains make an
angle " ! 28.0° with the vertical. (a) What is the speed of
each seat? (b) Draw a free-body diagram of a 40.0-kg child
riding in a seat and find the tension in the chain.

64.

A piece of putty is initially located at point A on the rim of
a  grinding  wheel  rotating  about  a  horizontal  axis.  The
putty  is  dislodged  from  point  A when  the  diameter
through A is horizontal. It then rises vertically and returns
to  A at  the  instant  the  wheel  completes  one  revolution.

Problems

179

66.

An example of the Coriolis effect. Suppose air resistance is negli-
gible for a golf ball. A golfer tees off from a location
precisely at 1

35.0° north latitude. He hits the ball due

south, with range 285 m. The ball’s initial velocity is at 48.0°
above the horizontal. (a) For how long is the ball in flight?
The cup is due south of the golfer’s location, and he would
have a hole-in-one if the Earth were not rotating. The
Earth’s rotation makes the tee move in a circle of ra-
dius R

cos 1

(6.37 ) 10

m) cos 35.0°, as shown in Fig-

ure P6.66. The tee completes one revolution each day.
(b) Find the eastward speed of the tee, relative to the stars.
The hole is also moving east, but it is 285 m farther south,
and thus at a slightly lower latitude 1

. Because the hole

moves  in  a  slightly  larger  circle,  its  speed  must  be  greater
than that of the tee. (c) By how much does the hole’s speed
exceed that of the tee? During the time the ball is in flight,
it  moves  upward  and  downward  as  well  as  southward  with
the projectile motion you studied in Chapter 4, but it also
moves eastward with the speed you found in part (b). The
hole  moves  to  the  east  at  a  faster  speed,  however,  pulling
ahead of the ball with the relative speed you found in part
(c). (d) How far to the west of the hole does the ball land?

67.

A car rounds a banked curve as in Figure 6.6. The radius
of curvature of the road is R, the banking angle is ", and
the coefficient of static friction is #

. (a) Determine the

range of speeds the car can have without slipping up or
down the road. (b) Find the minimum value for #

such

that the minimum speed is zero. (c) What is the range of
speeds possible if R ! 100 m, " ! 10.0°, and #

0.100

(slippery conditions)?

68.

A  single  bead  can  slide  with  negligible  friction  on  a  wire
that  is  bent  into  a  circular  loop  of  radius  15.0 cm,  as  in
Figure P6.68. The circle is always in a vertical plane and ro-
tates steadily about its vertical diameter with (a) a period
of 0.450 s. The position of the bead is described by the an-
gle " that the radial line, from the center of the loop to the
bead,  makes  with  the  vertical.  At  what  angle  up  from  the
bottom of the circle can the bead stay motionless relative

to the turning circle? (b) What If? Repeat the problem if
the period of the circle’s rotation is 0.850 s.

69.

The expression F ! arv ( br

gives the magnitude of the

resistive force (in newtons) exerted on a sphere of radius r
(in meters) by a stream of air moving at speed v (in meters
per second), where a and b are constants with appropriate
SI units. Their numerical values are a ! 3.10 ) 10

and

b ! 0.870.  Using  this  expression,  find  the  terminal  speed
for water droplets falling under their own weight in air, tak-
ing  the  following  values  for  the  drop  radii:  (a)  10.0 #m,
(b) 100 #m,  (c)  1.00 mm.  Note  that  for  (a)  and  (c)  you
can  obtain  accurate  answers  without  solving  a  quadratic
equation,  by  considering  which  of  the  two  contributions
to the air  resistance  is  dominant  and  ignoring  the  lesser
contribution.

70.

A  9.00-kg  object  starting  from  rest  falls  through  a  viscous
medium and experiences a resistive force R ! ' bv, where
v is the velocity of the object. If the object reaches one-half
its  terminal  speed  in  5.54 s,  (a)  determine  the  terminal
speed.  (b)  At  what  time  is  the  speed  of  the  object  three-
fourths  the  terminal  speed?  (c)  How  far  has  the  object
traveled in the first 5.54 s of motion?
A  model  airplane  of  mass  0.750 kg  flies  in  a  horizontal
circle at the end of a 60.0-m control wire, with a speed of
35.0 m/s.  Compute  the  tension  in  the  wire  if  it  makes  a
constant  angle  of  20.0° with  the  horizontal.  The  forces
exerted  on  the  airplane  are  the  pull  of  the  control  wire,
the gravitational force, and aerodynamic lift, which acts at
20.0° inward from the vertical as shown in Figure P6.71.

71.

cos

Golf ball

trajectory

Figure P6.66

Figure P6.68

20.0

lift

Figure P6.71

180

CHAPTE R 6 • Circular Motion and Other Applications of Newton’s Laws

72.

Members of a skydiving club were given the following

data to use in planning their jumps. In the table, d is the
distance fallen from rest by a sky diver in a “free-fall stable
spread position,” versus the time of fall t. (a) Convert the
distances in feet into meters. (b) Graph d (in meters)
versus t. (c) Determine the value of the terminal speed v

by finding the slope of the straight portion of the curve.
Use a least-squares fit to determine this slope.

t (s)

d (ft)

t (s)

d (ft)

1 309

1 483

138

1 657

242

1 831

366

2 005

504

2 179

652

2 353

808

2 527

971

2 701

1 138

2 875

73.

If a single constant force acts on an object that moves on a
straight line, the object’s velocity is a linear function of
time. The equation v ! v

(

at gives its velocity v as a func-

tion  of  time,  where  a is  its  constant  acceleration.  What  if
velocity  is  instead  a  linear  function  of  position?  Assume
that  as  a  particular  object  moves  through  a  resistive
medium, its speed decreases as described by the equation
v ! v

kx, where k is a constant coefficient and x is the

position of the object. Find the law describing the total
force acting on this object.

Answers to Quick Quizzes

6.1 (b), (d). The centripetal acceleration is always toward the

center of the circular path.

6.2 (a), (d). The normal force is always perpendicular to the

surface that applies the force. Because your car maintains
its orientation at all points on the ride, the normal force is
always upward.

6.3 (a). If the car is moving in a circular path, it must have

centripetal acceleration given by Equation 4.15.

6.4 Because the speed is constant, the only direction the

force can have is that of the centripetal acceleration.
The force is larger at # than at ! because the radius at
#

is smaller. There is no force at " because the wire is

straight.

6.5 In addition to the forces in the centripetal direction in

Quick Quiz 6.4, there are now tangential forces to provide
the tangential acceleration. The tangential force is the
same at all three points because the tangential accelera-
tion is constant.

6.6 (c). The only forces acting on the passenger are the con-

tact force with the door and the friction force from the
seat. Both of these are real forces and both act to the left
in Figure 6.11. Fictitious forces should never be drawn in a
force diagram.

6.7 (a). The basketball, having a larger cross-sectional area,

will have a larger force due to air resistance than the base-
ball. This will result in a smaller net force in the downward
direction and a smaller downward acceleration.

Content .. 43 44 45 46 ..